Difference between revisions of "Directory:Jon Awbrey/Papers/Dynamics And Logic"
Jon Awbrey (talk | contribs) (→Note 4: convert graphics) |
Jon Awbrey (talk | contribs) (add cats) |
||
| (9 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
{{DISPLAYTITLE:Dynamics And Logic}} | {{DISPLAYTITLE:Dynamics And Logic}} | ||
| + | |||
| + | '''Note.''' Many problems with the sucky MathJax on this page. The parser apparently reads 4 tildes inside math brackets the way it would in the external wiki environment, in other words, as signature tags. [[User:Jon Awbrey|Jon Awbrey]] ([[User talk:Jon Awbrey|talk]]) 18:00, 5 December 2014 (UTC) | ||
| + | |||
==Note 1== | ==Note 1== | ||
| Line 13: | Line 16: | ||
Let us start with a proposition of the form <math>p ~\operatorname{and}~ q</math> that is graphed as two labels attached to a root node: | Let us start with a proposition of the form <math>p ~\operatorname{and}~ q</math> that is graphed as two labels attached to a root node: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph Existential P And Q.jpg|500px]] | | [[Image:Cactus Graph Existential P And Q.jpg|500px]] | ||
|} | |} | ||
| Line 23: | Line 26: | ||
In this style of graphical representation, the value <math>\operatorname{true}</math> looks like a blank label and the value <math>\operatorname{false}</math> looks like an edge. | In this style of graphical representation, the value <math>\operatorname{true}</math> looks like a blank label and the value <math>\operatorname{false}</math> looks like an edge. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph Existential True.jpg|500px]] | | [[Image:Cactus Graph Existential True.jpg|500px]] | ||
|} | |} | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph Existential False.jpg|500px]] | | [[Image:Cactus Graph Existential False.jpg|500px]] | ||
|} | |} | ||
| Line 33: | Line 36: | ||
Back to the proposition <math>pq.\!</math> Imagine yourself standing in a fixed cell of the corresponding venn diagram, say, the cell where the proposition <math>pq\!</math> is true, as shown in the following Figure: | Back to the proposition <math>pq.\!</math> Imagine yourself standing in a fixed cell of the corresponding venn diagram, say, the cell where the proposition <math>pq\!</math> is true, as shown in the following Figure: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Venn Diagram P And Q.jpg|500px]] | | [[Image:Venn Diagram P And Q.jpg|500px]] | ||
|} | |} | ||
| Line 41: | Line 44: | ||
Don't think about it — just compute: | Don't think about it — just compute: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph (P,dP)(Q,dQ).jpg|500px]] | | [[Image:Cactus Graph (P,dP)(Q,dQ).jpg|500px]] | ||
|} | |} | ||
| Line 47: | Line 50: | ||
The cactus formula <math>\texttt{(p, dp)(q, dq)}</math> and its corresponding graph arise by substituting <math>p + \operatorname{d}p</math> for <math>p\!</math> and <math>q + \operatorname{d}q</math> for <math>q\!</math> in the boolean product or logical conjunction <math>pq\!</math> and writing the result in the two dialects of cactus syntax. This follows from the fact that the boolean sum <math>p + \operatorname{d}p</math> is equivalent to the logical operation of exclusive disjunction, which parses to a cactus graph of the following form: | The cactus formula <math>\texttt{(p, dp)(q, dq)}</math> and its corresponding graph arise by substituting <math>p + \operatorname{d}p</math> for <math>p\!</math> and <math>q + \operatorname{d}q</math> for <math>q\!</math> in the boolean product or logical conjunction <math>pq\!</math> and writing the result in the two dialects of cactus syntax. This follows from the fact that the boolean sum <math>p + \operatorname{d}p</math> is equivalent to the logical operation of exclusive disjunction, which parses to a cactus graph of the following form: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph (P,dP).jpg|500px]] | | [[Image:Cactus Graph (P,dP).jpg|500px]] | ||
|} | |} | ||
| Line 54: | Line 57: | ||
proposition <math>pq\!</math> over there, at a distance of <math>\operatorname{d}p</math> and <math>\operatorname{d}q,</math> and the value of the proposition <math>pq\!</math> where you are standing, all expressed in the form of a general formula, of course? Here is the appropriate formulation: | proposition <math>pq\!</math> over there, at a distance of <math>\operatorname{d}p</math> and <math>\operatorname{d}q,</math> and the value of the proposition <math>pq\!</math> where you are standing, all expressed in the form of a general formula, of course? Here is the appropriate formulation: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph ((P,dP)(Q,dQ),PQ).jpg|500px]] | | [[Image:Cactus Graph ((P,dP)(Q,dQ),PQ).jpg|500px]] | ||
|} | |} | ||
| Line 62: | Line 65: | ||
Last question, for now: What is the value of this expression from your current standpoint, that is, evaluated at the point where <math>pq\!</math> is true? Well, substituting <math>1\!</math> for <math>p\!</math> and <math>1\!</math> for <math>q\!</math> in the graph amounts to erasing the labels <math>p\!</math> and <math>q\!,</math> as shown here: | Last question, for now: What is the value of this expression from your current standpoint, that is, evaluated at the point where <math>pq\!</math> is true? Well, substituting <math>1\!</math> for <math>p\!</math> and <math>1\!</math> for <math>q\!</math> in the graph amounts to erasing the labels <math>p\!</math> and <math>q\!,</math> as shown here: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph (( ,dP)( ,dQ), ).jpg|500px]] | | [[Image:Cactus Graph (( ,dP)( ,dQ), ).jpg|500px]] | ||
|} | |} | ||
| Line 68: | Line 71: | ||
And this is equivalent to the following graph: | And this is equivalent to the following graph: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph ((dP)(dQ)).jpg|500px]] | | [[Image:Cactus Graph ((dP)(dQ)).jpg|500px]] | ||
|} | |} | ||
| Line 76: | Line 79: | ||
We have just met with the fact that the differential of the '''''and''''' is the '''''or''''' of the differentials. | We have just met with the fact that the differential of the '''''and''''' is the '''''or''''' of the differentials. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center; width:90%" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 84: | Line 87: | ||
& \quad & | & \quad & | ||
\operatorname{d}p ~\operatorname{or}~ \operatorname{d}q | \operatorname{d}p ~\operatorname{or}~ \operatorname{d}q | ||
| − | \end{matrix}</math> | + | \end{matrix}\!</math> |
|} | |} | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph PQ Diff ((dP)(dQ)).jpg|500px]] | | [[Image:Cactus Graph PQ Diff ((dP)(dQ)).jpg|500px]] | ||
|} | |} | ||
| Line 99: | Line 102: | ||
We begin with a proposition or a boolean function <math>f(p, q) = pq.\!</math> | We begin with a proposition or a boolean function <math>f(p, q) = pq.\!</math> | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Venn Diagram F = P And Q.jpg|500px]] | | [[Image:Venn Diagram F = P And Q.jpg|500px]] | ||
|- | |- | ||
| Line 107: | Line 110: | ||
A function like this has an abstract type and a concrete type. The abstract type is what we invoke when we write things like <math>f : \mathbb{B} \times \mathbb{B} \to \mathbb{B}</math> or <math>f : \mathbb{B}^2 \to \mathbb{B}.</math> The concrete type takes into account the qualitative dimensions or the "units" of the case, which can be explained as follows. | A function like this has an abstract type and a concrete type. The abstract type is what we invoke when we write things like <math>f : \mathbb{B} \times \mathbb{B} \to \mathbb{B}</math> or <math>f : \mathbb{B}^2 \to \mathbb{B}.</math> The concrete type takes into account the qualitative dimensions or the "units" of the case, which can be explained as follows. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" width="90%" |
| Let <math>P\!</math> be the set of values <math>\{ \texttt{(} p \texttt{)},~ p \} ~=~ \{ \operatorname{not}~ p,~ p \} ~\cong~ \mathbb{B}.</math> | | Let <math>P\!</math> be the set of values <math>\{ \texttt{(} p \texttt{)},~ p \} ~=~ \{ \operatorname{not}~ p,~ p \} ~\cong~ \mathbb{B}.</math> | ||
|- | |- | ||
| Line 119: | Line 122: | ||
The first couple of operators that we need to consider are logical analogues of the pair that play a founding role in the classical finite difference calculus, namely: | The first couple of operators that we need to consider are logical analogues of the pair that play a founding role in the classical finite difference calculus, namely: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" width="90%" |
| The ''difference operator'' <math>\Delta,\!</math> written here as <math>\operatorname{D}.</math> | | The ''difference operator'' <math>\Delta,\!</math> written here as <math>\operatorname{D}.</math> | ||
|- | |- | ||
| Line 129: | Line 132: | ||
In order to describe the universe in which these operators operate, it is necessary to enlarge the original universe of discourse. Starting from the initial space <math>X = P \times Q,</math> its ''(first order) differential extension'' <math>\operatorname{E}X</math> is constructed according to the following specifications: | In order to describe the universe in which these operators operate, it is necessary to enlarge the original universe of discourse. Starting from the initial space <math>X = P \times Q,</math> its ''(first order) differential extension'' <math>\operatorname{E}X</math> is constructed according to the following specifications: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{array}{rcc} | <math>\begin{array}{rcc} | ||
\operatorname{E}X & = & X \times \operatorname{d}X | \operatorname{E}X & = & X \times \operatorname{d}X | ||
| − | \end{array}</math> | + | \end{array}\!</math> |
|} | |} | ||
where: | where: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{array}{rcc} | <math>\begin{array}{rcc} | ||
| Line 156: | Line 159: | ||
& = & | & = & | ||
\{ \texttt{(} \operatorname{d}q \texttt{)},~ \operatorname{d}q \} | \{ \texttt{(} \operatorname{d}q \texttt{)},~ \operatorname{d}q \} | ||
| − | \end{array}</math> | + | \end{array}\!</math> |
|} | |} | ||
| − | The interpretations of these new symbols can be diverse, but the easiest | + | The interpretations of these new symbols can be diverse, but the easiest option for now is just to say that <math>\operatorname{d}p\!</math> means "change <math>p\!</math>" and <math>\operatorname{d}q</math> means "change <math>q\!</math>". |
| − | option for now is just to say that <math>\operatorname{d}p</math> means "change <math>p\!</math>" and <math>\operatorname{d}q</math> means "change <math>q\!</math>". | ||
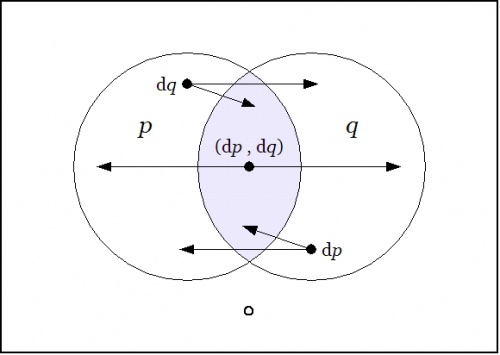
Drawing a venn diagram for the differential extension <math>\operatorname{E}X = X \times \operatorname{d}X</math> requires four logical dimensions, <math>P, Q, \operatorname{d}P, \operatorname{d}Q,</math> but it is possible to project a suggestion of what the differential features <math>\operatorname{d}p</math> and <math>\operatorname{d}q</math> are about on the 2-dimensional base space <math>X = P \times Q</math> by drawing arrows that cross the boundaries of the basic circles in the venn diagram for <math>X\!,</math> reading an arrow as <math>\operatorname{d}p</math> if it crosses the boundary between <math>p\!</math> and <math>\texttt{(} p \texttt{)}</math> in either direction and reading an arrow as <math>\operatorname{d}q</math> if it crosses the boundary between <math>q\!</math> and <math>\texttt{(} q \texttt{)}</math> in either direction. | Drawing a venn diagram for the differential extension <math>\operatorname{E}X = X \times \operatorname{d}X</math> requires four logical dimensions, <math>P, Q, \operatorname{d}P, \operatorname{d}Q,</math> but it is possible to project a suggestion of what the differential features <math>\operatorname{d}p</math> and <math>\operatorname{d}q</math> are about on the 2-dimensional base space <math>X = P \times Q</math> by drawing arrows that cross the boundaries of the basic circles in the venn diagram for <math>X\!,</math> reading an arrow as <math>\operatorname{d}p</math> if it crosses the boundary between <math>p\!</math> and <math>\texttt{(} p \texttt{)}</math> in either direction and reading an arrow as <math>\operatorname{d}q</math> if it crosses the boundary between <math>q\!</math> and <math>\texttt{(} q \texttt{)}</math> in either direction. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Venn Diagram P Q dP dQ.jpg|500px]] | | [[Image:Venn Diagram P Q dP dQ.jpg|500px]] | ||
|} | |} | ||
| Line 175: | Line 177: | ||
following formula: | following formula: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 188: | Line 190: | ||
In the example <math>f(p, q) = pq,\!</math> the enlargement <math>\operatorname{E}f</math> is computed as follows: | In the example <math>f(p, q) = pq,\!</math> the enlargement <math>\operatorname{E}f</math> is computed as follows: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 203: | Line 205: | ||
Given the proposition <math>f(p, q)\!</math> over <math>X = P \times Q,</math> the ''(first order) difference'' of <math>f\!</math> is the proposition <math>\operatorname{D}f</math> over <math>\operatorname{E}X</math> that is defined by the formula <math>\operatorname{D}f = \operatorname{E}f - f,</math> or, written out in full: | Given the proposition <math>f(p, q)\!</math> over <math>X = P \times Q,</math> the ''(first order) difference'' of <math>f\!</math> is the proposition <math>\operatorname{D}f</math> over <math>\operatorname{E}X</math> that is defined by the formula <math>\operatorname{D}f = \operatorname{E}f - f,</math> or, written out in full: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 216: | Line 218: | ||
In the example <math>f(p, q) = pq,\!</math> the difference <math>\operatorname{D}f</math> is computed as follows: | In the example <math>f(p, q) = pq,\!</math> the difference <math>\operatorname{D}f</math> is computed as follows: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 231: | Line 233: | ||
We did not yet go through the trouble to interpret this (first order) ''difference of conjunction'' fully, but were happy simply to evaluate it with respect to a single location in the universe of discourse, namely, at the point picked out by the singular proposition <math>pq,\!</math> that is, at the place where <math>p = 1\!</math> and <math>q = 1.\!</math> This evaluation is written in the form <math>\operatorname{D}f|_{pq}</math> or <math>\operatorname{D}f|_{(1, 1)},</math> and we arrived at the locally applicable law that is stated and illustrated as follows: | We did not yet go through the trouble to interpret this (first order) ''difference of conjunction'' fully, but were happy simply to evaluate it with respect to a single location in the universe of discourse, namely, at the point picked out by the singular proposition <math>pq,\!</math> that is, at the place where <math>p = 1\!</math> and <math>q = 1.\!</math> This evaluation is written in the form <math>\operatorname{D}f|_{pq}</math> or <math>\operatorname{D}f|_{(1, 1)},</math> and we arrived at the locally applicable law that is stated and illustrated as follows: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>f(p, q) ~=~ pq ~=~ p ~\operatorname{and}~ q \quad \Rightarrow \quad \operatorname{D}f|_{pq} ~=~ \texttt{((} \operatorname{dp} \texttt{)(} \operatorname{d}q \texttt{))} ~=~ \operatorname{d}p ~\operatorname{or}~ \operatorname{d}q</math> | <math>f(p, q) ~=~ pq ~=~ p ~\operatorname{and}~ q \quad \Rightarrow \quad \operatorname{D}f|_{pq} ~=~ \texttt{((} \operatorname{dp} \texttt{)(} \operatorname{d}q \texttt{))} ~=~ \operatorname{d}p ~\operatorname{or}~ \operatorname{d}q</math> | ||
| Line 242: | Line 244: | ||
The picture shows the analysis of the inclusive disjunction <math>\texttt{((} \operatorname{d}p \texttt{)(} \operatorname{d}q \texttt{))}</math> into the following exclusive disjunction: | The picture shows the analysis of the inclusive disjunction <math>\texttt{((} \operatorname{d}p \texttt{)(} \operatorname{d}q \texttt{))}</math> into the following exclusive disjunction: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 265: | Line 267: | ||
In the example <math>f(p, q) = pq,\!</math> the value of the difference proposition <math>\operatorname{D}f_x</math> at each of the four points in <math>x \in X\!</math> may be computed in graphical fashion as shown below: | In the example <math>f(p, q) = pq,\!</math> the value of the difference proposition <math>\operatorname{D}f_x</math> at each of the four points in <math>x \in X\!</math> may be computed in graphical fashion as shown below: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph Df = ((P,dP)(Q,dQ),PQ).jpg|500px]] | | [[Image:Cactus Graph Df = ((P,dP)(Q,dQ),PQ).jpg|500px]] | ||
|- | |- | ||
| Line 279: | Line 281: | ||
The easy way to visualize the values of these graphical expressions is just to notice the following equivalents: | The easy way to visualize the values of these graphical expressions is just to notice the following equivalents: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Cactus Graph Lobe Rule.jpg|500px]] | | [[Image:Cactus Graph Lobe Rule.jpg|500px]] | ||
|- | |- | ||
| Line 287: | Line 289: | ||
Laying out the arrows on the augmented venn diagram, one gets a picture of a ''differential vector field''. | Laying out the arrows on the augmented venn diagram, one gets a picture of a ''differential vector field''. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| [[Image:Venn Diagram PQ Difference Conj.jpg|500px]] | | [[Image:Venn Diagram PQ Difference Conj.jpg|500px]] | ||
|} | |} | ||
| Line 293: | Line 295: | ||
The Figure shows the points of the extended universe <math>\operatorname{E}X = P \times Q \times \operatorname{d}P \times \operatorname{d}Q</math> that are indicated by the difference map <math>\operatorname{D}f : \operatorname{E}X \to \mathbb{B},</math> namely, the following six points or singular propositions:: | The Figure shows the points of the extended universe <math>\operatorname{E}X = P \times Q \times \operatorname{d}P \times \operatorname{d}Q</math> that are indicated by the difference map <math>\operatorname{D}f : \operatorname{E}X \to \mathbb{B},</math> namely, the following six points or singular propositions:: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" |
| | | | ||
<math>\begin{array}{rcccc} | <math>\begin{array}{rcccc} | ||
| Line 318: | Line 320: | ||
Abstracting from the augmented venn diagram that shows how the ''models'' or ''satisfying interpretations'' of <math>\operatorname{D}f</math> distribute over the extended universe of discourse <math>\operatorname{E}X = P \times Q \times \operatorname{d}P \times \operatorname{d}Q,</math> the difference map <math>\operatorname{D}f</math> can be represented in the form of a ''digraph'' or ''directed graph'', one whose points are labeled with the elements of <math>X = P \times Q</math> and whose arrows are labeled with the elements of <math>\operatorname{d}X = \operatorname{d}P \times \operatorname{d}Q,</math> as shown in the following Figure. | Abstracting from the augmented venn diagram that shows how the ''models'' or ''satisfying interpretations'' of <math>\operatorname{D}f</math> distribute over the extended universe of discourse <math>\operatorname{E}X = P \times Q \times \operatorname{d}P \times \operatorname{d}Q,</math> the difference map <math>\operatorname{D}f</math> can be represented in the form of a ''digraph'' or ''directed graph'', one whose points are labeled with the elements of <math>X = P \times Q</math> and whose arrows are labeled with the elements of <math>\operatorname{d}X = \operatorname{d}P \times \operatorname{d}Q,</math> as shown in the following Figure. | ||
| − | {| align="center" cellpadding="10" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Directed Graph PQ Difference Conj.jpg|500px]] | | [[Image:Directed Graph PQ Difference Conj.jpg|500px]] | ||
| − | | | + | |- |
| − | |||
| − | |||
| | | | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
| Line 347: | Line 347: | ||
A suitably generic definition of the extended universe of discourse is afforded by the following set-up: | A suitably generic definition of the extended universe of discourse is afforded by the following set-up: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{array}{lccl} | <math>\begin{array}{lccl} | ||
| Line 372: | Line 372: | ||
For a proposition of the form <math>f : X_1 \times \ldots \times X_k \to \mathbb{B},</math> the ''(first order) enlargement'' of <math>f\!</math> is the proposition <math>\operatorname{E}f : \operatorname{E}X \to \mathbb{B}</math> that is defined by the following equation: | For a proposition of the form <math>f : X_1 \times \ldots \times X_k \to \mathbb{B},</math> the ''(first order) enlargement'' of <math>f\!</math> is the proposition <math>\operatorname{E}f : \operatorname{E}X \to \mathbb{B}</math> that is defined by the following equation: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{array}{l} | <math>\begin{array}{l} | ||
| Line 387: | Line 387: | ||
In the example of logical conjunction, <math>f(p, q) = pq,\!</math> the enlargement <math>\operatorname{E}f</math> is formulated as follows: | In the example of logical conjunction, <math>f(p, q) = pq,\!</math> the enlargement <math>\operatorname{E}f</math> is formulated as follows: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{array}{l} | <math>\begin{array}{l} | ||
| Line 400: | Line 400: | ||
Given that this expression uses nothing more than the boolean ring operations of addition and multiplication, it is permissible to "multiply things out" in the usual manner to arrive at the following result: | Given that this expression uses nothing more than the boolean ring operations of addition and multiplication, it is permissible to "multiply things out" in the usual manner to arrive at the following result: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 417: | Line 417: | ||
To understand what the ''enlarged'' or ''shifted'' proposition means in logical terms, it serves to go back and analyze the above expression for <math>\operatorname{E}f</math> in the same way that we did for <math>\operatorname{D}f.</math> Toward that end, the value of <math>\operatorname{E}f_x</math> at each <math>x \in X</math> may be computed in graphical fashion as shown below: | To understand what the ''enlarged'' or ''shifted'' proposition means in logical terms, it serves to go back and analyze the above expression for <math>\operatorname{E}f</math> in the same way that we did for <math>\operatorname{D}f.</math> Toward that end, the value of <math>\operatorname{E}f_x</math> at each <math>x \in X</math> may be computed in graphical fashion as shown below: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" style="text-align:center" |
| − | + | | [[Image:Cactus Graph Ef = (P,dP)(Q,dQ).jpg|500px]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | Ef = | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | [[Image:Cactus Graph Ef@PQ = (dP)(dQ).jpg|500px]] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | [[Image:Cactus Graph Ef@P(Q) = (dP)dQ.jpg|500px]] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | [[Image:Cactus Graph Ef@(P)Q = dP(dQ).jpg|500px]] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | [[Image:Cactus Graph Ef@(P)(Q) = dP dQ.jpg|500px]] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
Given the data that develops in this form of analysis, the disjoined ingredients can now be folded back into a boolean expansion or a disjunctive normal form (DNF) that is equivalent to the enlarged proposition <math>\operatorname{E}f.</math> | Given the data that develops in this form of analysis, the disjoined ingredients can now be folded back into a boolean expansion or a disjunctive normal form (DNF) that is equivalent to the enlarged proposition <math>\operatorname{E}f.</math> | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" width="90%" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 521: | Line 448: | ||
Here is a summary of the result, illustrated by means of a digraph picture, where the "no change" element <math>(\operatorname{d}p)(\operatorname{d}q)</math> is drawn as a loop at the point <math>p~q.</math> | Here is a summary of the result, illustrated by means of a digraph picture, where the "no change" element <math>(\operatorname{d}p)(\operatorname{d}q)</math> is drawn as a loop at the point <math>p~q.</math> | ||
| − | {| align="center" cellpadding="10" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Directed Graph PQ Enlargement Conj.jpg|500px]] | | [[Image:Directed Graph PQ Enlargement Conj.jpg|500px]] | ||
| − | | | + | |- |
| − | |||
| − | |||
| | | | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
| Line 649: | Line 574: | ||
(p)~q~ | (p)~q~ | ||
\\[4pt] | \\[4pt] | ||
| − | (p) | + | (p)[[User:Jon Awbrey|Jon Awbrey]] ([[User talk:Jon Awbrey|talk]]) |
\\[4pt] | \\[4pt] | ||
~p~(q) | ~p~(q) | ||
\\[4pt] | \\[4pt] | ||
| − | + | [[User:Jon Awbrey|Jon Awbrey]] ([[User talk:Jon Awbrey|talk]])(q) | |
\\[4pt] | \\[4pt] | ||
(p,~q) | (p,~q) | ||
| Line 756: | Line 681: | ||
((p,~q)) | ((p,~q)) | ||
\\[4pt] | \\[4pt] | ||
| − | + | 17:54, 5 December 2014 (UTC)q~~ | |
\\[4pt] | \\[4pt] | ||
~(p~(q)) | ~(p~(q)) | ||
\\[4pt] | \\[4pt] | ||
| − | ~~ | + | ~~p17:54, 5 December 2014 (UTC) |
\\[4pt] | \\[4pt] | ||
((p)~q)~ | ((p)~q)~ | ||
| Line 2,708: | Line 2,633: | ||
|} | |} | ||
| − | For example, given the set <math>X = \{ a, b, c \},\!</math> suppose that we have the 2-adic relative term <math>\mathit{m} = {}^{\backprime\backprime}\, \text{marker for}\, \underline{ | + | For example, given the set <math>X = \{ a, b, c \},\!</math> suppose that we have the 2-adic relative term <math>\mathit{m} = {}^{\backprime\backprime}\, \text{marker for}\, \underline{[[User:Jon Awbrey|Jon Awbrey]] ([[User talk:Jon Awbrey|talk]]) 17:54, 5 December 2014 (UTC)}\, {}^{\prime\prime}</math> and |
the associated 2-adic relation <math>M \subseteq X \times X,</math> the general pattern of whose common structure is represented by the following matrix: | the associated 2-adic relation <math>M \subseteq X \times X,</math> the general pattern of whose common structure is represented by the following matrix: | ||
| Line 2,808: | Line 2,733: | ||
|} | |} | ||
| − | Recognizing that <math>a\!:\!a + b\!:\!b + c\!:\!c</math> is the identity transformation otherwise known as <math>\mathit{1},\!</math> the 2-adic relative term <math>m = {}^{\backprime\backprime}\, \text{marker for}\, \underline{ | + | Recognizing that <math>a\!:\!a + b\!:\!b + c\!:\!c</math> is the identity transformation otherwise known as <math>\mathit{1},\!</math> the 2-adic relative term <math>m = {}^{\backprime\backprime}\, \text{marker for}\, \underline{[[User:Jon Awbrey|Jon Awbrey]] ([[User talk:Jon Awbrey|talk]]) 17:54, 5 December 2014 (UTC)}\, {}^{\prime\prime}</math> can be parsed as an element <math>\mathit{1} + a\!:\!b + b\!:\!c + c\!:\!a</math> of the so-called ''group ring'', all of which makes this element just a special sort of linear transformation. |
Up to this point, we are still reading the elementary relatives of the form <math>i\!:\!j</math> in the way that Peirce read them in logical contexts: <math>i\!</math> is the relate, <math>j\!</math> is the correlate, and in our current example <math>i\!:\!j,</math> or more exactly, <math>m_{ij} = 1,\!</math> is taken to say that <math>i\!</math> is a marker for <math>j.\!</math> This is the mode of reading that we call "multiplying on the left". | Up to this point, we are still reading the elementary relatives of the form <math>i\!:\!j</math> in the way that Peirce read them in logical contexts: <math>i\!</math> is the relate, <math>j\!</math> is the correlate, and in our current example <math>i\!:\!j,</math> or more exactly, <math>m_{ij} = 1,\!</math> is taken to say that <math>i\!</math> is a marker for <math>j.\!</math> This is the mode of reading that we call "multiplying on the left". | ||
| Line 3,048: | Line 2,973: | ||
Here is the operation table for <math>S_3,\!</math> given in abstract fashion: | Here is the operation table for <math>S_3,\!</math> given in abstract fashion: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" style="text-align:center" |
| − | + | | <math>\text{Symmetric Group}~ S_3</math> | |
| − | < | + | |- |
| − | Symmetric Group S_3 | + | | [[Image:Symmetric Group S(3).jpg|500px]] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
| Line 3,145: | Line 3,028: | ||
To construct the regular representations of <math>S_3,\!</math> we begin with the data of its operation table: | To construct the regular representations of <math>S_3,\!</math> we begin with the data of its operation table: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" style="text-align:center" |
| − | + | | <math>\text{Symmetric Group}~ S_3</math> | |
| − | < | + | |- |
| − | Symmetric Group S_3 | + | | [[Image:Symmetric Group S(3).jpg|500px]] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
| Line 3,199: | Line 3,040: | ||
Since we have a function of the type <math>L : G \times G \to G,</math> we can define a couple of substitution operators: | Since we have a function of the type <math>L : G \times G \to G,</math> we can define a couple of substitution operators: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" width="90%" |
| valign="top" | 1. | | valign="top" | 1. | ||
| <math>\operatorname{Sub}(x, (\underline{~~}, y))</math> puts any specified <math>x\!</math> into the empty slot of the rheme <math>(\underline{~~}, y),</math> with the effect of producing the saturated rheme <math>(x, y)\!</math> that evaluates to <math>xy.\!</math> | | <math>\operatorname{Sub}(x, (\underline{~~}, y))</math> puts any specified <math>x\!</math> into the empty slot of the rheme <math>(\underline{~~}, y),</math> with the effect of producing the saturated rheme <math>(x, y)\!</math> that evaluates to <math>xy.\!</math> | ||
| Line 3,209: | Line 3,050: | ||
In (1) we consider the effects of each <math>x\!</math> in its practical bearing on contexts of the form <math>(\underline{~~}, y),</math> as <math>y\!</math> ranges over <math>G,\!</math> and the effects are such that <math>x\!</math> takes <math>(\underline{~~}, y)</math> into <math>xy,\!</math> for <math>y\!</math> in <math>G,\!</math> all of which is notated as <math>x = \{ (y : xy) ~|~ y \in G \}.</math> The pairs <math>(y : xy)\!</math> can be found by picking an <math>x\!</math> from the left margin of the group operation table and considering its effects on each <math>y\!</math> in turn as these run along the right margin. This produces the ''regular ante-representation'' of <math>S_3,\!</math> like so: | In (1) we consider the effects of each <math>x\!</math> in its practical bearing on contexts of the form <math>(\underline{~~}, y),</math> as <math>y\!</math> ranges over <math>G,\!</math> and the effects are such that <math>x\!</math> takes <math>(\underline{~~}, y)</math> into <math>xy,\!</math> for <math>y\!</math> in <math>G,\!</math> all of which is notated as <math>x = \{ (y : xy) ~|~ y \in G \}.</math> The pairs <math>(y : xy)\!</math> can be found by picking an <math>x\!</math> from the left margin of the group operation table and considering its effects on each <math>y\!</math> in turn as these run along the right margin. This produces the ''regular ante-representation'' of <math>S_3,\!</math> like so: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" style="text-align:center" |
| − | + | | | |
<math>\begin{array}{*{13}{c}} | <math>\begin{array}{*{13}{c}} | ||
\operatorname{e} | \operatorname{e} | ||
| Line 3,264: | Line 3,105: | ||
In (2) we consider the effects of each <math>x\!</math> in its practical bearing on contexts of the form <math>(y, \underline{~~}),</math> as <math>y\!</math> ranges over <math>G,\!</math> and the effects are such that <math>x\!</math> takes <math>(y, \underline{~~})</math> into <math>yx,\!</math> for <math>y\!</math> in <math>G,\!</math> all of which is notated as <math>x = \{ (y : yx) ~|~ y \in G \}.</math> The pairs <math>(y : yx)\!</math> can be found by picking an <math>x\!</math> on the right margin of the group operation table and considering its effects on each <math>y\!</math> in turn as these run along the left margin. This produces the ''regular post-representation'' of <math>S_3,\!</math> like so: | In (2) we consider the effects of each <math>x\!</math> in its practical bearing on contexts of the form <math>(y, \underline{~~}),</math> as <math>y\!</math> ranges over <math>G,\!</math> and the effects are such that <math>x\!</math> takes <math>(y, \underline{~~})</math> into <math>yx,\!</math> for <math>y\!</math> in <math>G,\!</math> all of which is notated as <math>x = \{ (y : yx) ~|~ y \in G \}.</math> The pairs <math>(y : yx)\!</math> can be found by picking an <math>x\!</math> on the right margin of the group operation table and considering its effects on each <math>y\!</math> in turn as these run along the left margin. This produces the ''regular post-representation'' of <math>S_3,\!</math> like so: | ||
| − | {| align="center" cellpadding=" | + | {| align="center" cellpadding="10" style="text-align:center" |
| − | + | | | |
<math>\begin{array}{*{13}{c}} | <math>\begin{array}{*{13}{c}} | ||
\operatorname{e} | \operatorname{e} | ||
| Line 3,543: | Line 3,384: | ||
Let us take a moment to view an old proposition in this new light, for example, the logical conjunction <math>pq : X \to \mathbb{B}</math> pictured in Figure 22-a. | Let us take a moment to view an old proposition in this new light, for example, the logical conjunction <math>pq : X \to \mathbb{B}</math> pictured in Figure 22-a. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,557: | Line 3,398: | ||
The field of changes produced by <math>\operatorname{E}</math> on <math>pq\!</math> is shown in Figure 22-b. | The field of changes produced by <math>\operatorname{E}</math> on <math>pq\!</math> is shown in Figure 22-b. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Enlargement Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Enlargement Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,603: | Line 3,444: | ||
The field of changes produced by <math>\operatorname{D}\!</math> on <math>pq\!</math> is shown in Figure 22-c. | The field of changes produced by <math>\operatorname{D}\!</math> on <math>pq\!</math> is shown in Figure 22-c. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Difference Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Difference Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,663: | Line 3,504: | ||
A generic enough picture at this stage of the game, and one that will remind us of these fundamental features of the cybernetic system even as things get far more complex, is afforded by Figure 23. | A generic enough picture at this stage of the game, and one that will remind us of these fundamental features of the cybernetic system even as things get far more complex, is afforded by Figure 23. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center; width:90%" |
| | | | ||
<pre> | <pre> | ||
| Line 3,677: | Line 3,518: | ||
| / \ | | | / \ | | ||
| / \ | | | / \ | | ||
| − | | o | + | | o G o | |
| | | | | | | | | | ||
| | | | | | | | | | ||
| | | | | | | | | | ||
| − | | | | + | | | o<---------T---------o | |
| | | | | | | | | | ||
| | | | | | | | | | ||
| Line 3,688: | Line 3,529: | ||
| \ / | | | \ / | | ||
| \ / | | | \ / | | ||
| − | | \ | + | | \ / | |
| − | | \ | + | | \ / | |
| \ / | | | \ / | | ||
| \ / | | | \ / | | ||
| Line 3,709: | Line 3,550: | ||
Figure 24-1 shows the proposition <math>pq\!</math> once again, which we now view as a scalar field — analogous to a ''potential hill'' in physics, but in logic tantamount to a ''potential plateau'' — where the shaded region indicates an elevation of 1 and the unshaded region indicates an elevation of 0. | Figure 24-1 shows the proposition <math>pq\!</math> once again, which we now view as a scalar field — analogous to a ''potential hill'' in physics, but in logic tantamount to a ''potential plateau'' — where the shaded region indicates an elevation of 1 and the unshaded region indicates an elevation of 0. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,719: | Line 3,560: | ||
Figure 24-2 shows the tacit extension of the scalar field <math>pq : X \to \mathbb{B}</math> to the differential field <math>\varepsilon (pq) : \operatorname{E}X \to \mathbb{B}.</math> | Figure 24-2 shows the tacit extension of the scalar field <math>pq : X \to \mathbb{B}</math> to the differential field <math>\varepsilon (pq) : \operatorname{E}X \to \mathbb{B}.</math> | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Tacit Extension Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Tacit Extension Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,753: | Line 3,594: | ||
Continuing with the example <math>pq : X \to \mathbb{B},</math> Figure 25-1 shows the enlargement or shift map <math>\operatorname{E}(pq) : \operatorname{E}X \to \mathbb{B}</math> in the same style of differential field picture that we drew for the tacit extension <math>\varepsilon (pq) : \operatorname{E}X \to \mathbb{B}.</math> | Continuing with the example <math>pq : X \to \mathbb{B},</math> Figure 25-1 shows the enlargement or shift map <math>\operatorname{E}(pq) : \operatorname{E}X \to \mathbb{B}</math> in the same style of differential field picture that we drew for the tacit extension <math>\varepsilon (pq) : \operatorname{E}X \to \mathbb{B}.</math> | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Enlargement Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Enlargement Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,799: | Line 3,640: | ||
In this case one notices that the tacit extension <math>\varepsilon f</math> and the enlargement <math>\operatorname{E}f</math> are in a certain sense dual to each other. The tacit extension <math>\varepsilon f</math> indicates all the arrows out of the region where <math>f\!</math> is true and the enlargement <math>\operatorname{E}f</math> indicates all the arrows into the region where <math>f\!</math> is true. The only arc they have in common is the no-change loop <math>\texttt{(} \operatorname{d}p \texttt{)(} \operatorname{d}q \texttt{)}</math> at <math>pq.\!</math> If we add the two sets of arcs in mod 2 fashion then the loop of multiplicity 2 zeroes out, leaving the 6 arrows of <math>\operatorname{D}(pq) = \varepsilon(pq) + \operatorname{E}(pq)</math> that are illustrated in Figure 25-2. | In this case one notices that the tacit extension <math>\varepsilon f</math> and the enlargement <math>\operatorname{E}f</math> are in a certain sense dual to each other. The tacit extension <math>\varepsilon f</math> indicates all the arrows out of the region where <math>f\!</math> is true and the enlargement <math>\operatorname{E}f</math> indicates all the arrows into the region where <math>f\!</math> is true. The only arc they have in common is the no-change loop <math>\texttt{(} \operatorname{d}p \texttt{)(} \operatorname{d}q \texttt{)}</math> at <math>pq.\!</math> If we add the two sets of arcs in mod 2 fashion then the loop of multiplicity 2 zeroes out, leaving the 6 arrows of <math>\operatorname{D}(pq) = \varepsilon(pq) + \operatorname{E}(pq)</math> that are illustrated in Figure 25-2. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Difference Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Difference Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,855: | Line 3,696: | ||
Figure 26-1 shows the differential proposition <math>\operatorname{d}f = \operatorname{d}(pq) : \operatorname{E}X \to \mathbb{B}</math> that we get by extracting the cell-wise linear approximation to the difference map <math>\operatorname{D}f = \operatorname{D}(pq) : \operatorname{E}X \to \mathbb{B}.</math> This is the logical analogue of what would ordinarily be called ''the'' differential of <math>pq,\!</math> but since I've been attaching the adjective ''differential'' to just about everything in sight, the distinction tends to be lost. For the time being, I'll resort to using the alternative name ''tangent map'' for <math>\operatorname{d}f.\!</math> | Figure 26-1 shows the differential proposition <math>\operatorname{d}f = \operatorname{d}(pq) : \operatorname{E}X \to \mathbb{B}</math> that we get by extracting the cell-wise linear approximation to the difference map <math>\operatorname{D}f = \operatorname{D}(pq) : \operatorname{E}X \to \mathbb{B}.</math> This is the logical analogue of what would ordinarily be called ''the'' differential of <math>pq,\!</math> but since I've been attaching the adjective ''differential'' to just about everything in sight, the distinction tends to be lost. For the time being, I'll resort to using the alternative name ''tangent map'' for <math>\operatorname{d}f.\!</math> | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Differential Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Differential Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,863: | Line 3,704: | ||
Just to be clear about what's being indicated here, it's a visual way of summarizing the following data: | Just to be clear about what's being indicated here, it's a visual way of summarizing the following data: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
| Line 3,886: | Line 3,727: | ||
To understand the extended interpretations, that is, the conjunctions of basic and differential features that are being indicated here, it may help to note the following equivalences: | To understand the extended interpretations, that is, the conjunctions of basic and differential features that are being indicated here, it may help to note the following equivalences: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Line 3,921: | Line 3,762: | ||
Capping the series that analyzes the proposition <math>pq\!</math> in terms of succeeding orders of linear propositions, Figure 26-2 shows the remainder map <math>\operatorname{r}(pq) : \operatorname{E}X \to \mathbb{B},</math> that happens to be linear in pairs of variables. | Capping the series that analyzes the proposition <math>pq\!</math> in terms of succeeding orders of linear propositions, Figure 26-2 shows the remainder map <math>\operatorname{r}(pq) : \operatorname{E}X \to \mathbb{B},</math> that happens to be linear in pairs of variables. | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| [[Image:Field Picture PQ Remainder Conjunction.jpg|500px]] | | [[Image:Field Picture PQ Remainder Conjunction.jpg|500px]] | ||
|- | |- | ||
| Line 3,929: | Line 3,770: | ||
Reading the arrows off the map produces the following data: | Reading the arrows off the map produces the following data: | ||
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center" |
| | | | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
| Line 4,051: | Line 3,892: | ||
# http://forum.wolframscience.com/showthread.php?postid=1602#post1602 | # http://forum.wolframscience.com/showthread.php?postid=1602#post1602 | ||
# http://forum.wolframscience.com/showthread.php?postid=1603#post1603 | # http://forum.wolframscience.com/showthread.php?postid=1603#post1603 | ||
| + | |||
| + | [[Category:Artificial Intelligence]] | ||
| + | [[Category:Boolean Algebra]] | ||
| + | [[Category:Boolean Functions]] | ||
| + | [[Category:Charles Sanders Peirce]] | ||
| + | [[Category:Combinatorics]] | ||
| + | [[Category:Computational Complexity]] | ||
| + | [[Category:Computer Science]] | ||
| + | [[Category:Cybernetics]] | ||
| + | [[Category:Differential Logic]] | ||
| + | [[Category:Equational Reasoning]] | ||
| + | [[Category:Formal Languages]] | ||
| + | [[Category:Formal Systems]] | ||
| + | [[Category:Graph Theory]] | ||
| + | [[Category:Inquiry]] | ||
| + | [[Category:Inquiry Driven Systems]] | ||
| + | [[Category:Knowledge Representation]] | ||
| + | [[Category:Logic]] | ||
| + | [[Category:Logical Graphs]] | ||
| + | [[Category:Mathematics]] | ||
| + | [[Category:Philosophy]] | ||
| + | [[Category:Propositional Calculus]] | ||
| + | [[Category:Semiotics]] | ||
| + | [[Category:Visualization]] | ||
Latest revision as of 13:28, 10 December 2014
Note. Many problems with the sucky MathJax on this page. The parser apparently reads 4 tildes inside math brackets the way it would in the external wiki environment, in other words, as signature tags. Jon Awbrey (talk) 18:00, 5 December 2014 (UTC)
Note 1
I am going to excerpt some of my previous explorations on differential logic and dynamic systems and bring them to bear on the sorts of discrete dynamical themes that we find of interest in the NKS Forum. This adaptation draws on the "Cactus Rules", "Propositional Equation Reasoning Systems", and "Reductions Among Relations" threads, and will in time be applied to the "Differential Analytic Turing Automata" thread:
One of the first things that you can do, once you have a moderately efficient calculus for boolean functions or propositional logic, whatever you choose to call it, is to start thinking about, and even start computing, the differentials of these functions or propositions.
Let us start with a proposition of the form \(p ~\operatorname{and}~ q\) that is graphed as two labels attached to a root node:

|
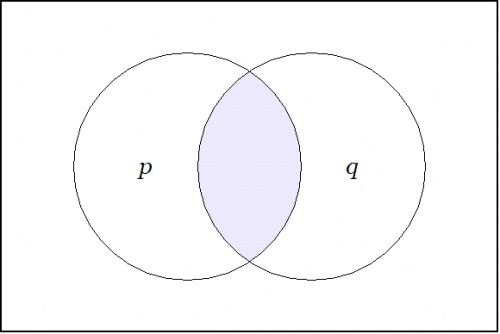
Written as a string, this is just the concatenation \(p~q\).
The proposition \(pq\!\) may be taken as a boolean function \(f(p, q)\!\) having the abstract type \(f : \mathbb{B} \times \mathbb{B} \to \mathbb{B},\) where \(\mathbb{B} = \{ 0, 1 \}\) is read in such a way that \(0\!\) means \(\operatorname{false}\) and \(1\!\) means \(\operatorname{true}.\)
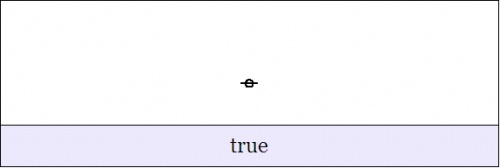
In this style of graphical representation, the value \(\operatorname{true}\) looks like a blank label and the value \(\operatorname{false}\) looks like an edge.
|
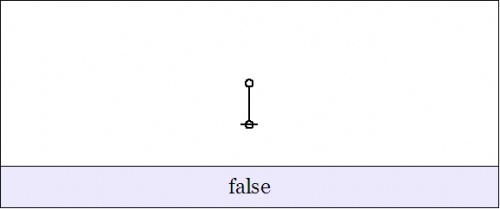
|
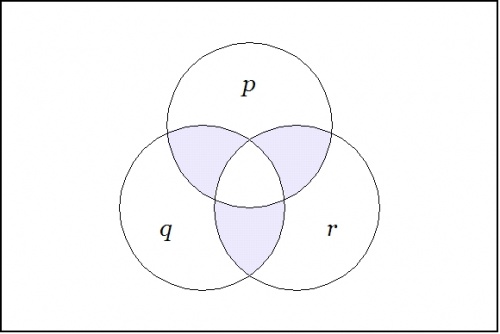
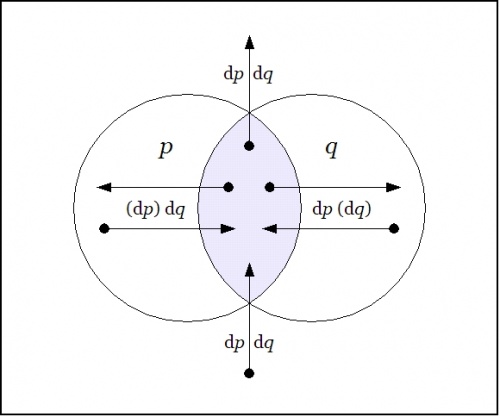
Back to the proposition \(pq.\!\) Imagine yourself standing in a fixed cell of the corresponding venn diagram, say, the cell where the proposition \(pq\!\) is true, as shown in the following Figure:
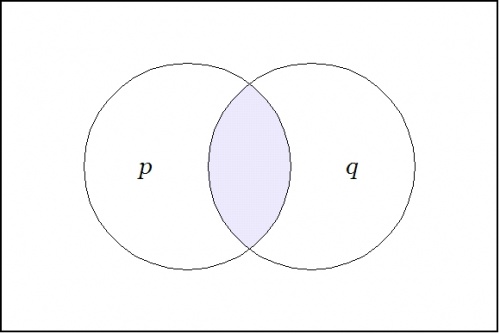
|
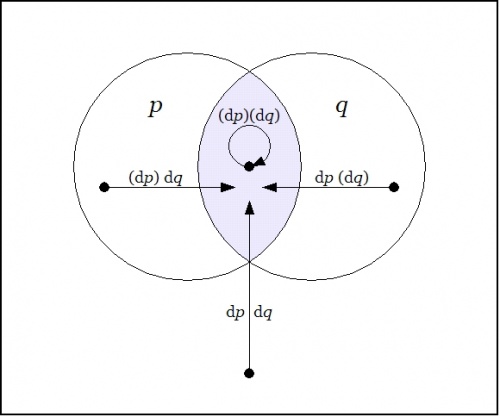
Now ask yourself: What is the value of the proposition \(pq\!\) at a distance of \(\operatorname{d}p\) and \(\operatorname{d}q\) from the cell \(pq\!\) where you are standing?
Don't think about it — just compute:
|
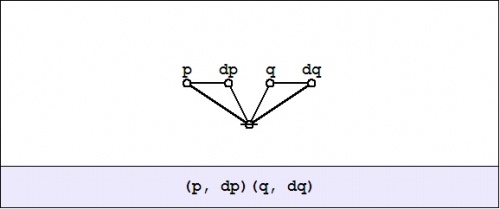
The cactus formula \(\texttt{(p, dp)(q, dq)}\) and its corresponding graph arise by substituting \(p + \operatorname{d}p\) for \(p\!\) and \(q + \operatorname{d}q\) for \(q\!\) in the boolean product or logical conjunction \(pq\!\) and writing the result in the two dialects of cactus syntax. This follows from the fact that the boolean sum \(p + \operatorname{d}p\) is equivalent to the logical operation of exclusive disjunction, which parses to a cactus graph of the following form:
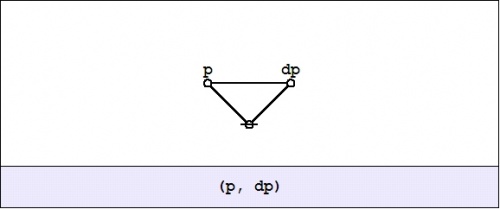
|
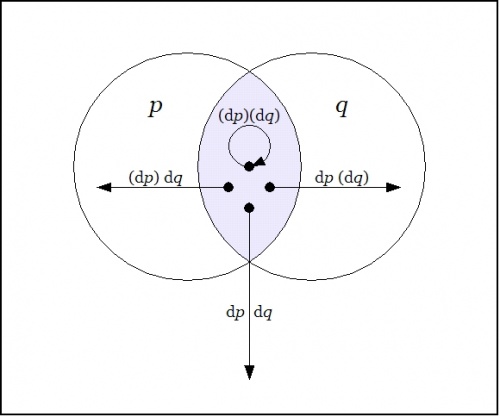
Next question: What is the difference between the value of the proposition \(pq\!\) over there, at a distance of \(\operatorname{d}p\) and \(\operatorname{d}q,\) and the value of the proposition \(pq\!\) where you are standing, all expressed in the form of a general formula, of course? Here is the appropriate formulation:
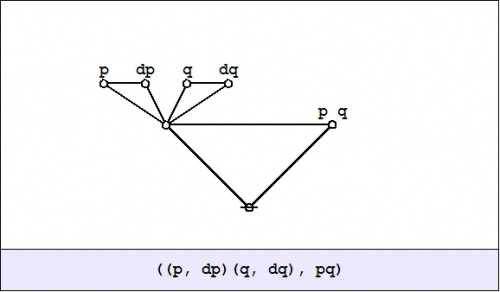
|
There is one thing that I ought to mention at this point: Computed over \(\mathbb{B},\) plus and minus are identical operations. This will make the relation between the differential and the integral parts of the appropriate calculus slightly stranger than usual, but we will get into that later.
Last question, for now: What is the value of this expression from your current standpoint, that is, evaluated at the point where \(pq\!\) is true? Well, substituting \(1\!\) for \(p\!\) and \(1\!\) for \(q\!\) in the graph amounts to erasing the labels \(p\!\) and \(q\!,\) as shown here:
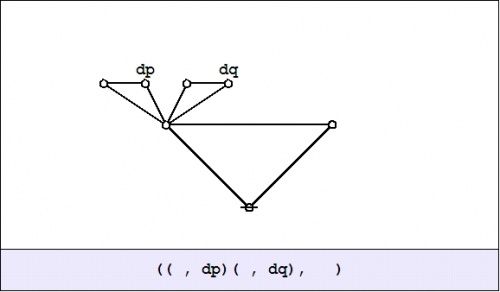
|
And this is equivalent to the following graph:
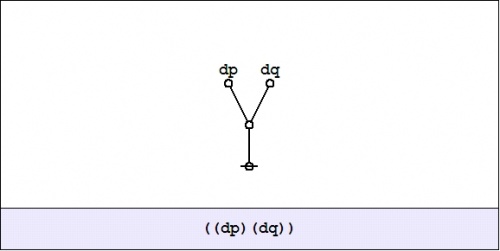
|
Note 2
We have just met with the fact that the differential of the and is the or of the differentials.
|
\(\begin{matrix} p ~\operatorname{and}~ q & \quad & \xrightarrow{\quad\operatorname{Diff}\quad} & \quad & \operatorname{d}p ~\operatorname{or}~ \operatorname{d}q \end{matrix}\!\) |
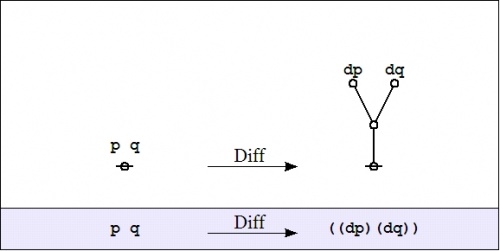
|
It will be necessary to develop a more refined analysis of that statement directly, but that is roughly the nub of it.
If the form of the above statement reminds you of De Morgan's rule, it is no accident, as differentiation and negation turn out to be closely related operations. Indeed, one can find discussions of logical difference calculus in the Boole–De Morgan correspondence and Peirce also made use of differential operators in a logical context, but the exploration of these ideas has been hampered by a number of factors, not the least of which has been the lack of a syntax that was adequate to handle the complexity of expressions that evolve.
Let us run through the initial example again, this time attempting to interpret the formulas that develop at each stage along the way.
We begin with a proposition or a boolean function \(f(p, q) = pq.\!\)

|

|
A function like this has an abstract type and a concrete type. The abstract type is what we invoke when we write things like \(f : \mathbb{B} \times \mathbb{B} \to \mathbb{B}\) or \(f : \mathbb{B}^2 \to \mathbb{B}.\) The concrete type takes into account the qualitative dimensions or the "units" of the case, which can be explained as follows.
| Let \(P\!\) be the set of values \(\{ \texttt{(} p \texttt{)},~ p \} ~=~ \{ \operatorname{not}~ p,~ p \} ~\cong~ \mathbb{B}.\) |
| Let \(Q\!\) be the set of values \(\{ \texttt{(} q \texttt{)},~ q \} ~=~ \{ \operatorname{not}~ q,~ q \} ~\cong~ \mathbb{B}.\) |
Then interpret the usual propositions about \(p, q\!\) as functions of the concrete type \(f : P \times Q \to \mathbb{B}.\)
We are going to consider various operators on these functions. Here, an operator \(\operatorname{F}\) is a function that takes one function \(f\!\) into another function \(\operatorname{F}f.\)
The first couple of operators that we need to consider are logical analogues of the pair that play a founding role in the classical finite difference calculus, namely:
| The difference operator \(\Delta,\!\) written here as \(\operatorname{D}.\) |
| The enlargement" operator \(\Epsilon,\!\) written here as \(\operatorname{E}.\) |
These days, \(\operatorname{E}\) is more often called the shift operator.
In order to describe the universe in which these operators operate, it is necessary to enlarge the original universe of discourse. Starting from the initial space \(X = P \times Q,\) its (first order) differential extension \(\operatorname{E}X\) is constructed according to the following specifications:
|
\(\begin{array}{rcc} \operatorname{E}X & = & X \times \operatorname{d}X \end{array}\!\) |
where:
|
\(\begin{array}{rcc} X & = & P \times Q \'"`UNIQ-MathJax1-QINU`"' Amazing! =='"`UNIQ--h-10--QINU`"'Note 11== We have been contemplating functions of the type \(f : X \to \mathbb{B}\) and studying the action of the operators \(\operatorname{E}\) and \(\operatorname{D}\) on this family. These functions, that we may identify for our present aims with propositions, inasmuch as they capture their abstract forms, are logical analogues of scalar potential fields. These are the sorts of fields that are so picturesquely presented in elementary calculus and physics textbooks by images of snow-covered hills and parties of skiers who trek down their slopes like least action heroes. The analogous scene in propositional logic presents us with forms more reminiscent of plateaunic idylls, being all plains at one of two levels, the mesas of verity and falsity, as it were, with nary a niche to inhabit between them, restricting our options for a sporting gradient of downhill dynamics to just one of two: standing still on level ground or falling off a bluff. We are still working well within the logical analogue of the classical finite difference calculus, taking in the novelties that the logical transmutation of familiar elements is able to bring to light. Soon we will take up several different notions of approximation relationships that may be seen to organize the space of propositions, and these will allow us to define several different forms of differential analysis applying to propositions. In time we will find reason to consider more general types of maps, having concrete types of the form \(X_1 \times \ldots \times X_k \to Y_1 \times \ldots \times Y_n\) and abstract types \(\mathbb{B}^k \to \mathbb{B}^n.\) We will think of these mappings as transforming universes of discourse into themselves or into others, in short, as transformations of discourse. Before we continue with this intinerary, however, I would like to highlight another sort of differential aspect that concerns the boundary operator or the marked connective that serves as one of the two basic connectives in the cactus language for ZOL. For example, consider the proposition \(f\!\) of concrete type \(f : P \times Q \times R \to \mathbb{B}\) and abstract type \(f : \mathbb{B}^3 \to \mathbb{B}\) that is written \(\texttt{(} p, q, r \texttt{)}\) in cactus syntax. Taken as an assertion in what Peirce called the existential interpretation, the proposition \(\texttt{(} p, q, r \texttt{)}\) says that just one of \(p, q, r\!\) is false. It is instructive to consider this assertion in relation to the logical conjunction \(pqr\!\) of the same propositions. A venn diagram of \(\texttt{(} p, q, r \texttt{)}\) looks like this:
In relation to the center cell indicated by the conjunction \(pqr,\!\) the region indicated by \(\texttt{(} p, q, r \texttt{)}\) is comprised of the adjacent or bordering cells. Thus they are the cells that are just across the boundary of the center cell, reached as if by way of Leibniz's minimal changes from the point of origin, in this case, \(pqr.\!\) More generally speaking, in a \(k\!\)-dimensional universe of discourse that is based on the alphabet of features \(\mathcal{X} = \{ x_1, \ldots, x_k \},\) the same form of boundary relationship is manifested for any cell of origin that one chooses to indicate. One way to indicate a cell is by forming a logical conjunction of positive and negative basis features, that is, by constructing an expression of the form \(e_1 \cdot \ldots \cdot e_k,\) where \(e_j = x_j ~\text{or}~ e_j = \texttt{(} x_j \texttt{)},\) for \(j = 1 ~\text{to}~ k.\) The proposition \(\texttt{(} e_1, \ldots, e_k \texttt{)}\) indicates the disjunctive region consisting of the cells that are just next door to \(e_1 \cdot \ldots \cdot e_k.\) Note 12
One other subject that it would be opportune to mention at this point, while we have an object example of a mathematical group fresh in mind, is the relationship between the pragmatic maxim and what are commonly known in mathematics as representation principles. As it turns out, with regard to its formal characteristics, the pragmatic maxim unites the aspects of a representation principle with the attributes of what would ordinarily be known as a closure principle. We will consider the form of closure that is invoked by the pragmatic maxim on another occasion, focusing here and now on the topic of group representations. Let us return to the example of the four-group \(V_4.\!\) We encountered this group in one of its concrete representations, namely, as a transformation group that acts on a set of objects, in this case a set of sixteen functions or propositions. Forgetting about the set of objects that the group transforms among themselves, we may take the abstract view of the group's operational structure, for example, in the form of the group operation table copied here:
This table is abstractly the same as, or isomorphic to, the versions with the \(\operatorname{E}_{ij}\) operators and the \(\operatorname{T}_{ij}\) transformations that we took up earlier. That is to say, the story is the same, only the names have been changed. An abstract group can have a variety of significantly and superficially different representations. But even after we have long forgotten the details of any particular representation there is a type of concrete representations, called regular representations, that are always readily available, as they can be generated from the mere data of the abstract operation table itself. To see how a regular representation is constructed from the abstract operation table, select a group element from the top margin of the Table, and "consider its effects" on each of the group elements as they are listed along the left margin. We may record these effects as Peirce usually did, as a logical aggregate of elementary dyadic relatives, that is, as a logical disjunction or boolean sum whose terms represent the ordered pairs of \(\operatorname{input} : \operatorname{output}\) transactions that are produced by each group element in turn. This forms one of the two possible regular representations of the group, in this case the one that is called the post-regular representation or the right regular representation. It has long been conventional to organize the terms of this logical aggregate in the form of a matrix: Reading "\(+\!\)" as a logical disjunction:
And so, by expanding effects, we get:
|
- Artificial Intelligence
- Boolean Algebra
- Boolean Functions
- Charles Sanders Peirce
- Combinatorics
- Computational Complexity
- Computer Science
- Cybernetics
- Differential Logic
- Equational Reasoning
- Formal Languages
- Formal Systems
- Graph Theory
- Inquiry
- Inquiry Driven Systems
- Knowledge Representation
- Logic
- Logical Graphs
- Mathematics
- Philosophy
- Propositional Calculus
- Semiotics
- Visualization