Difference between revisions of "Directory:Jon Awbrey/Papers/Riffs and Rotes"
Jon Awbrey (talk | contribs) (→Idea) |
Jon Awbrey (talk | contribs) (→Idea) |
||
| Line 27: | Line 27: | ||
|} | |} | ||
| − | where <math>\text{p}_{i(k)}^{j(k)}</math> is the <math>k^\text{th}</math> prime power in the factorization and <math>\ell</math> is the number of distinct prime factors dividing <math>n.</math> | + | where <math>\text{p}_{i(k)}^{j(k)}</math> is the <math>k^\text{th}</math> prime power in the factorization and <math>\ell</math> is the number of distinct prime factors dividing <math>n.</math> The factorization of <math>1</math> is defined as <math>1</math> in accord with the convention that an empty product is equal to <math>1.</math> |
Let <math>I(n)</math> be the set of indices of primes that divide <math>n</math> and let <math>j(i, n)</math> be the number of times that <math>\text{p}_i</math> divides <math>n.</math> Then the prime factorization of <math>n</math> can be written in the following alternative form: | Let <math>I(n)</math> be the set of indices of primes that divide <math>n</math> and let <math>j(i, n)</math> be the number of times that <math>\text{p}_i</math> divides <math>n.</math> Then the prime factorization of <math>n</math> can be written in the following alternative form: | ||
| Line 42: | Line 42: | ||
9876543210 | 9876543210 | ||
& = & 2 \cdot 3^2 \cdot 5 \cdot {17}^2 \cdot 379721 | & = & 2 \cdot 3^2 \cdot 5 \cdot {17}^2 \cdot 379721 | ||
| − | & = & \text{p}_1^1 \text{p}_2^2 \text{p}_3^1 \text{p}_7^2 \text{p}_{32277}^1 | + | & = & \text{p}_1^1 \text{p}_2^2 \text{p}_3^1 \text{p}_7^2 \text{p}_{32277}^1. |
\end{matrix}</math> | \end{matrix}</math> | ||
|} | |} | ||
| + | |||
| + | Each index <math>i</math> and exponent <math>j</math> appearing in the prime factorization of a positive integer <math>n</math> is itself a positive integer, and thus has a prime factorization of its own. | ||
==Riffs in Numerical Order== | ==Riffs in Numerical Order== | ||
Revision as of 19:26, 3 February 2010
Idea
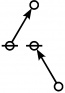
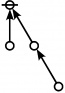
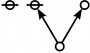
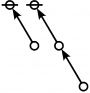
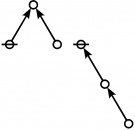
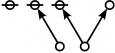
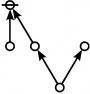
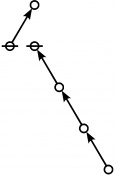
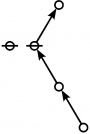
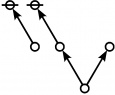
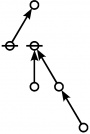
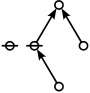
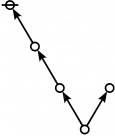
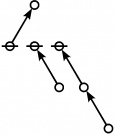
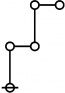
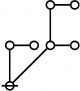
Let \(\text{p}_i\) be the \(i^\text{th}\) prime, where the positive integer \(i\) is called the index of the prime \(\text{p}_i\) and the indices are taken in such a way that \(\text{p}_1 = 2.\) Thus the sequence of primes begins as follows:
|
\(\begin{matrix} \text{p}_1 = 2, & \text{p}_2 = 3, & \text{p}_3 = 5, & \text{p}_4 = 7, & \text{p}_5 = 11, & \text{p}_6 = 13, & \text{p}_7 = 17, & \text{p}_8 = 19, & \ldots \end{matrix}\) |
The prime factorization of a positive integer \(n\) can be written in the following form:
| \(n ~=~ \prod_{k = 1}^{\ell} \text{p}_{i(k)}^{j(k)},\) |
where \(\text{p}_{i(k)}^{j(k)}\) is the \(k^\text{th}\) prime power in the factorization and \(\ell\) is the number of distinct prime factors dividing \(n.\) The factorization of \(1\) is defined as \(1\) in accord with the convention that an empty product is equal to \(1.\)
Let \(I(n)\) be the set of indices of primes that divide \(n\) and let \(j(i, n)\) be the number of times that \(\text{p}_i\) divides \(n.\) Then the prime factorization of \(n\) can be written in the following alternative form:
| \(n ~=~ \prod_{i \in I(n)} \text{p}_{i}^{j(i, n)}.\) |
For example:
|
\(\begin{matrix} 9876543210 & = & 2 \cdot 3^2 \cdot 5 \cdot {17}^2 \cdot 379721 & = & \text{p}_1^1 \text{p}_2^2 \text{p}_3^1 \text{p}_7^2 \text{p}_{32277}^1. \end{matrix}\) |
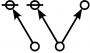
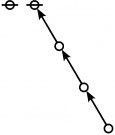
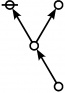
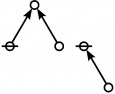
Each index \(i\) and exponent \(j\) appearing in the prime factorization of a positive integer \(n\) is itself a positive integer, and thus has a prime factorization of its own.
Riffs in Numerical Order
|
\(1\!\) \(\begin{array}{l} \varnothing \\ 1 \end{array}\) |
\(\text{p}\!\) \(\begin{array}{l} 1\!:\!1 \\ 2 \end{array}\) |
\(\text{p}_\text{p}\!\) \(\begin{array}{l} 2\!:\!1 \\ 3 \end{array}\) |
\(\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 \\ 4 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 \\ 5 \end{array}\) |
|
\(\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 \\ 6 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 4\!:\!1 \\ 7 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 \\ 8 \end{array}\) |
\(\text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 2\!:\!2 \\ 9 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!1 \\ 10 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 5\!:\!1 \\ 11 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 \\ 12 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 6\!:\!1 \\ 13 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 4\!:\!1 \\ 14 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 3\!:\!1 \\ 15 \end{array}\) |
|
\(\text{p}^{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!4 \\ 16 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 7\!:\!1 \\ 17 \end{array}\) |
\(\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!2 \\ 18 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 8\!:\!1 \\ 19 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 3\!:\!1 \\ 20 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 4\!:\!1 \\ 21 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 5\!:\!1 \\ 22 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 9\!:\!1 \\ 23 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 2\!:\!1 \\ 24 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 3\!:\!2 \\ 25 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 6\!:\!1 \\ 26 \end{array}\) |
\(\text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!3 \\ 27 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 4\!:\!1 \\ 28 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 10\!:\!1 \\ 29 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 30 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_{\text{p}_\text{p}}}}\!\) \(\begin{array}{l} 11\!:\!1 \\ 31 \end{array}\) |
\(\text{p}^{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!5 \\ 32 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 5\!:\!1 \\ 33 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 7\!:\!1 \\ 34 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 4\!:\!1 \\ 35 \end{array}\) |
|
\(\text{p}^\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!2 \\ 36 \end{array}\) |
\(\text{p}_{\text{p}^\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 12\!:\!1 \\ 37 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 8\!:\!1 \\ 38 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 6\!:\!1 \\ 39 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 3\!:\!1 \\ 40 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p} \text{p}_\text{p}}}\!\) \(\begin{array}{l} 13\!:\!1 \\ 41 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 4\!:\!1 \\ 42 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 14\!:\!1 \\ 43 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 5\!:\!1 \\ 44 \end{array}\) |
\(\text{p}_\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!2 ~~ 3\!:\!1 \\ 45 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 9\!:\!1 \\ 46 \end{array}\) |
\(\text{p}_{\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 15\!:\!1 \\ 47 \end{array}\) |
\(\text{p}^{\text{p}^\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!4 ~~ 2\!:\!1 \\ 48 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}^\text{p}\!\) \(\begin{array}{l} 4\!:\!2 \\ 49 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!2 \\ 50 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 7\!:\!1 \\ 51 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 6\!:\!1 \\ 52 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 16\!:\!1 \\ 53 \end{array}\) |
\(\text{p} \text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!3 \\ 54 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 5\!:\!1 \\ 55 \end{array}\) |
|
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 4\!:\!1 \\ 56 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 8\!:\!1 \\ 57 \end{array}\) |
\(\text{p} \text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 10\!:\!1 \\ 58 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}_{\text{p}^\text{p}}}}\!\) \(\begin{array}{l} 17\!:\!1 \\ 59 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 60 \end{array}\) |
Rotes in Numerical Order
|
\(1\!\) \(\begin{array}{l} \varnothing \\ 1 \end{array}\) |
\(\text{p}\!\) \(\begin{array}{l} 1\!:\!1 \\ 2 \end{array}\) |
\(\text{p}_\text{p}\!\) \(\begin{array}{l} 2\!:\!1 \\ 3 \end{array}\) |
\(\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 \\ 4 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 \\ 5 \end{array}\) |
|
\(\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 \\ 6 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 4\!:\!1 \\ 7 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 \\ 8 \end{array}\) |
\(\text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 2\!:\!2 \\ 9 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!1 \\ 10 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 5\!:\!1 \\ 11 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 \\ 12 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 6\!:\!1 \\ 13 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 4\!:\!1 \\ 14 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 3\!:\!1 \\ 15 \end{array}\) |
|
\(\text{p}^{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!4 \\ 16 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 7\!:\!1 \\ 17 \end{array}\) |
\(\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!2 \\ 18 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 8\!:\!1 \\ 19 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 3\!:\!1 \\ 20 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 4\!:\!1 \\ 21 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 5\!:\!1 \\ 22 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 9\!:\!1 \\ 23 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 2\!:\!1 \\ 24 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 3\!:\!2 \\ 25 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 6\!:\!1 \\ 26 \end{array}\) |
\(\text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!3 \\ 27 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 4\!:\!1 \\ 28 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 10\!:\!1 \\ 29 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 30 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_{\text{p}_\text{p}}}}\!\) \(\begin{array}{l} 11\!:\!1 \\ 31 \end{array}\) |
\(\text{p}^{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!5 \\ 32 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 5\!:\!1 \\ 33 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 7\!:\!1 \\ 34 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 4\!:\!1 \\ 35 \end{array}\) |
|
\(\text{p}^\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!2 \\ 36 \end{array}\) |
\(\text{p}_{\text{p}^\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 12\!:\!1 \\ 37 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 8\!:\!1 \\ 38 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 6\!:\!1 \\ 39 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 3\!:\!1 \\ 40 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p} \text{p}_\text{p}}}\!\) \(\begin{array}{l} 13\!:\!1 \\ 41 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 4\!:\!1 \\ 42 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 14\!:\!1 \\ 43 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 5\!:\!1 \\ 44 \end{array}\) |
\(\text{p}_\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!2 ~~ 3\!:\!1 \\ 45 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 9\!:\!1 \\ 46 \end{array}\) |
\(\text{p}_{\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 15\!:\!1 \\ 47 \end{array}\) |
\(\text{p}^{\text{p}^\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!4 ~~ 2\!:\!1 \\ 48 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}^\text{p}\!\) \(\begin{array}{l} 4\!:\!2 \\ 49 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!2 \\ 50 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 7\!:\!1 \\ 51 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 6\!:\!1 \\ 52 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 16\!:\!1 \\ 53 \end{array}\) |
\(\text{p} \text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!3 \\ 54 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 5\!:\!1 \\ 55 \end{array}\) |
|
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 4\!:\!1 \\ 56 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 8\!:\!1 \\ 57 \end{array}\) |
\(\text{p} \text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 10\!:\!1 \\ 58 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}_{\text{p}^\text{p}}}}\!\) \(\begin{array}{l} 17\!:\!1 \\ 59 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 60 \end{array}\) |
Selected Sequences
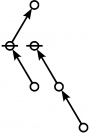
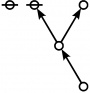
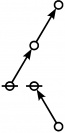
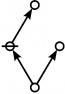
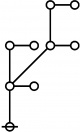
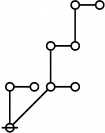
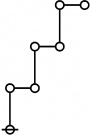
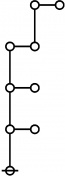
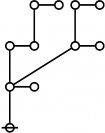
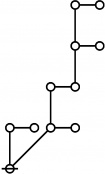
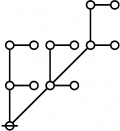
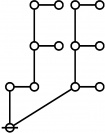
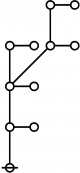
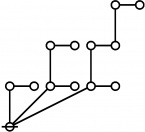
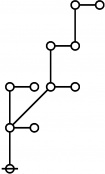
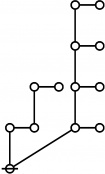
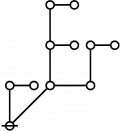
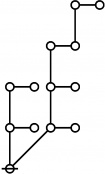
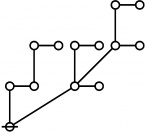
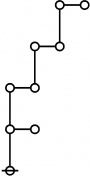
A061396
- Number of "rooted index-functional forests" (Riffs) on n nodes.
- Number of "rooted odd trees with only exponent symmetries" (Rotes) on 2n+1 nodes.